The logic of the probabilistic language

Home

Scientific project

Social project

Our story

Scientific community

Book index

Our network

Frisardi Masticationpedia Network

Fontana Masticationpedia Network

Ed. Lab

Donate

Contact

Change language

[[|German]]

[[|Spanish]]

[[|French]]

[[|Italian]]

The logic of the probabilistic language

Abstract

The text deals with the logic of probabilistic language applied to the medical field, highlighting how uncertainty is an intrinsic part of scientific practice. Through probabilistic and statistical concepts, efforts are made to manage and understand the uncertainties associated with medical theory and practice.

The role of probability in the relationship between theory and observation is emphasized, distinguishing between subjective uncertainty and randomness. Subjective uncertainty concerns individuals' state of knowledge and belief, while randomness refers to the lack of a certain connection between cause and effect.

In the medical approach, the importance of understanding and distinguishing between subjective and objective probability is discussed. Subjective probability reflects individual belief, while objective probability is based on data and empirical evidence.

The concept of probabilistic-causal analysis is then further explored, which seeks to quantify the relationship between events and random processes in clinical diagnosis. A detailed exposition is presented on how conditional probabilities can be interpreted and how causal relevance partitioning can be used to formulate a differential diagnosis.

Finally, the theme of interdisciplinarity in scientific research is addressed, highlighting the importance of an interdisciplinary approach to tackling complex problems. Fuzzy logic is also mentioned as a possible tool for managing uncertainty in medical contexts.

 

 

Every scientific idea—whether in medicine, architecture, engineering, chemistry, or any other field—when implemented, is prone to small errors and uncertainties. Mathematics, through the lens of probability theory and statistical inference, aids in precisely managing and thereby mitigating these uncertainties. It must always be considered that in all practical scenarios, "the outcomes also depend on many other external factors to the theory," be they initial and environmental conditions, experimental errors, or others.

The uncertainties surrounding these factors render the theory-observation relationship probabilistic. In medical practice, two types of uncertainty predominantly impact diagnoses: subjective uncertainty and causality. Therefore, in this context, it becomes crucial to differentiate between these two uncertainties and to demonstrate that the concept of probability assumes different meanings in these contexts. We will endeavor to elucidate these concepts by connecting each critical step to the clinical approach that has been documented in previous chapters, particularly focusing on the dental and neurological domains in vying for diagnostic supremacy for our dear Mary Poppins.

---

Subjective Uncertainty and Causality

Let's imagine asking Mary Poppins which of the two medical colleagues—the dentist or the neurologist—is correct.

The question would generate a kind of agitation based on internal uncertainty; therefore, the notions of certainty and uncertainty refer to the subjective epistemic states of human beings, and not to states of the external world, because there is no certainty or uncertainty in that world. In this sense, as we have mentioned, there are an inner world and an external world that both do not adhere to canons of uncertainty, but rather to probability.

Mary Poppins may be subjectively certain or uncertain as to whether she is suffering from TMDs or a neuropathic or neuromuscular form of OP. This is because "uncertainty" is a subjective, epistemic state below the threshold of knowledge and belief; hence the term.

Subjective Uncertainty

Without a doubt, the term ‘subjective’ alarms many, especially those who aim to practice science by pursuing the noble ideal of ‘objectivity,’ as this term is perceived by common sense. Therefore, it is appropriate to make some clarifications on the use of this term in this context:

‘Subjective’ indicates that the probability assessment depends on the information status of the individual who performs it.

‘Objective’ does not mean arbitrary.

The so-called ‘objectivity,’ as perceived by those outside scientific research, is achieved when a community of rational beings shares the same state of information. But even in this case, one should more properly speak of ‘intersubjectivity’ (i.e., the sharing of subjective opinions by a group).

In clinical cases—precisely because patients rarely possess advanced notions of medicine—subjective uncertainty must be considered. Living with uncertainty requires us to adopt a probabilistic approach.

Causality

Causality indicates the lack of a certain connection between cause and effect. The uncertainty of a close union between the source and the phenomenon is among the most challenging problems in determining a diagnosis.

In a clinical case, a phenomenon $A(x)$ (such as a malocclusion, a crossbite, an openbite, etc.) is randomly associated with another phenomenon $B(x)$ (such as TMJ bone degeneration); when there are exceptions for which the logical proposition $A(x)\rightarrow B(x)$ is not always true (but it is most of the time), we will say that the relation $A(x)\rightarrow B(x)$ is not always true but it is probable.

«We are moving from a deterministic condition to a stochastic one.»

Subjective and Objective Probability

In this chapter, we revisit some topics previously discussed in the insightful book by Kazem Sadegh-Zadeh, which tackles the problem of the logic of medical language. We adapt their content to our clinical case of Mary Poppins, aiming to keep our understanding more relevant to dental contexts.

Events that are both random and subjectively uncertain are considered probable; therefore, causality and uncertainty are approached as qualitative, comparative, or quantitative probabilities.

To clarify this concept, let's use the example of Mary Poppins. A doctor, after hearing her symptoms, could state that:

1. Mary Poppins is probably suffering from TMDs (qualitative term).
2. Mary Poppins is more likely to have TMDs than neuropathic OP (comparative term: the number of diagnosed cases of TMDs vs. neuropathic OP).
3. The probability that Mary Poppins has TMDs is 0.15 (quantitative term, relative to the population).

Subjective Probability

In contexts of human subjective uncertainty, probabilistic, qualitative, comparative, and/or quantitative data can be interpreted by clinicians as measures of subjective uncertainty, to make 'states of conviction' numerically representable.

For instance, stating "the probability that Mary Poppins is affected by TMDs is 0.15 of the cases" is akin to saying "with a 15% degree of belief, I think that Mary Poppins is affected by TMDs"; indicating that the degree of conviction corresponds to the degree of subjective probability.

Objective Probability

Conversely, events and random processes cannot be accurately described by deterministic processes in the form 'if A then B'. Statistics are utilized to quantify the frequency of the association between A and B, representing their relationship as a degree of probability, which introduces the concept of objective probability.

Amid the increasing acknowledgment of the role of probabilization of uncertainty and randomness in medicine since the eighteenth century, the term "probability" has become an integral part of medical language, methodology, and epistemology. Unfortunately, the distinction between subjective and objective probability is not always clearly made in medicine, as is the case in other disciplines too. Nevertheless, the vital contribution of probability theory in medicine, especially in concepts of probability in etiology, epidemiology, diagnostics, and therapy, lies in its aid in understanding and representing biological causality.

---

Probabilistic-Causal Analysis

From these premises, it is evident that clinical diagnosis employs the so-called hypothetical-deductive method, known as DN (deductive-nomological model). However, this is not realistic, as the medical knowledge used in clinical decision-making rarely contains causal deterministic laws that allow for causal explanations and, consequently, the formulation of clinical diagnoses, among other things in the specialist context. Let us re-examine the case of Mary Poppins, this time adopting a probabilistic-causal approach.

Consider a number $n$ of individuals, including those who report Orofacial Pain, who generally have bone degeneration of the Temporomandibular Joint. However, there might also be other apparently unrelated causes. We must mathematically translate the 'relevance' of these causal uncertainties in determining a diagnosis.

---

The casual relevance

To do this we consider the degree of causal relevance $(cr)$ of an event $E_{1}$ with respect to an event $E_{2}$ where:

• $E_{1}$ = patients with bone degeneration of the temporomandibular joint.

• $E_{2}$ = patients reporting orofacial pain.

• $E_{3}$ = patients without bone degeneration of the temporomandibular joint.

We will use the conditional probability $P(A\mid B)$, that is the probability that the $A$ event occurs only after the event $B$ has already occurred.

With these premises the causal relevance $cr$ of the sample $n$ of patients is:

$cr=P(E_{2}\mid E_{1})-P(E_{2}\mid E_{3})$

where

$P(E_{2}\mid E_{1})$ indicates the probability that some people (among $n$ taken into consideration) suffer from Orofacial Pain caused by bone degeneration of the Temporomandibular Joint,

while

$P(E_{2}\mid E_{3})$ indicates the probability that other people (always among $n$ taken into consideration) suffer from Orofacial Pain conditioned by something other than bone degeneration of the Temporomandibular Joint.

Since all probability suggest that $P(A\mid B)$ is a value between $0$ and $1$, the parameter $(cr)$ will be a number that is between $-1$ and $1$.

The meanings that we can give to this number are as follows:

• we have the extreme cases (which in reality never occur) which are:

• $cr=1$ indicating that the only cause of orofacial pain is bone degeneration of the TMJ,
• $cr=-1$ which indicates that the cause of orofacial pain is never bone degeneration of the TMJ but is something else,
• $cr=0$ indicating that the probability that orofacial pain is caused by bone degeneration of the TMJ or otherwise is exactly the same,

• and the intermediate cases (which are the realistic ones)

• $cr>0$ indicating that the cause of orofacial pain is more likely to be bone degeneration of the TMJ,
• $cr<0$ which indicates that the cause of orofacial pain is more likely not bone degeneration of the TMJ.

Second Clinical Approach

(hover over the images)

• 

  Figure 1: Patient reporting "Orofacial pain in the right hemilateral"

• 

  Figure 2: Patient's TMJ Stratigraphy showing signs of condylar flattening and osteophyte

• 

  Figure 3: Computed Tomography of the TMJ

• 

  Figure 4: Axiography of the patient showing a flattening of the chewing pattern on the right condyle

• 

  Figure 5: EMG Interferential Pattern. Overlapping upper traces corresponding to the right masseter, lower to the left masseter.

So be it then $P(D)$ the probability of finding, in the sample of our $n$ people, individuals who present the elements belonging to the aforementioned set $D=\{\delta _{1},\delta _{2},...,\delta _{n}\}$

In order to take advantage of the information provided by this dataset, the concept of partition of causal relevance is introduced:

The partition of causal relevance

Always be $n$ the number of people we have to conduct the analyses upon, if we divide (based on certain conditions as explained below) this group into $k$ subsets $C_{i}$ with $i=1,2,\dots ,k$, a cluster is created that is called a "partition set" $\pi$:

$\pi =\{C_{1},C_{2},\dots ,C_{k}\}\qquad \qquad {\text{with}}\qquad \qquad C_{i}\subset n,$

where with the symbolism $C_{i}\subset n$ it indicates that the subclass $C_{i}$ is contained in $n$.

The partition $\pi$, in order for it to be defined as a partition of causal relevance, must have these properties:

1. For each subclass $C_{i}$ the condition must apply $rc=P(D\mid C_{i})-P(D)\neq 0,$ ie the probability of finding in the subgroup $C_{i}$ a person who has the symptoms, clinical signs and elements belonging to the set $D=\{\delta _{1},\delta _{2},...,\delta _{n}\}$. A causally relevant partition of this type is said to be homogeneous.
2. Each subset $C_{i}$ must be 'elementary', i.e. it must not be further divided into other subsets, because if these existed they would have no causal relevance.

Now let us assume, for example, that the population sample $n$, to which our good patient Mary Poppins belongs, is a category of subjects aged 20 to 70. We also assume that in this population we have those who present the elements belonging to the data set $D=\{\delta _{1},.....\delta _{n}\}$ which correspond to the laboratory tests mentioned above and precisa in 'The logic of classical language'.

Let us suppose that in a sample of 10,000 subjects from 20 to 70 we will have an incidence of 30 subjects $p(D)=0.003$ showing clinical signs $\delta _{1}$ and $\delta _{4}$. We preferred to use these reports for the demonstration of the probabilistic process because in the literature the data regarding clinical signs and symptoms for Temporomandibular Disorders have too wide a variation as well as too high an incidence in our opinion.

An example of a partition with presumed probability in which TMJ degeneration (Deg.TMJ) occurs in conjunction with Temporomandibular Disorders (TMDs) would be the following:

$P(D|Deg.TMJ\cap TMDs)=0.95\qquad \qquad \;$		where			$\mathbb {C} _{1}\equiv Deg.TMJ\cap TMDs$
$P(D|Deg.TMJ\cap noTMDs)=0.3\qquad \qquad \quad$		where			$C_{2}\equiv Deg.TMJ\cap noTMDs$
$P(D|noDeg.TMJ\cap TMDs)=0.199\qquad \qquad \;$		where			$C_{3}\equiv noDeg.TMJ\cap TMDs$
$P(D|noDeg.TMJ\cap noTMDs)=0.001\qquad \qquad \;$		where			$C_{4}\equiv noDeg.TMJ\cap noTMDs$

• «A homogeneous partition provides what we are used to calling Differential Diagnosis.»

Clinical situations

These conditional probabilities demonstrate that each of the partition's four subclasses is causally relevant to patient data $D=\{\delta _{1},.....\delta _{n}\}$ in the population sample $PO$. Given the aforementioned partition of the reference class, we have the following clinical situations:

• Mary Poppins $\in$ degeneration of the temporomandibular joint $\cap$ Temporomandibular Disorders

• Mary Poppins $\in$ degeneration of the temporomandibular joint $\cap$ no Temporomandibular Disorders

• Mary Poppins $\in$ no degeneration of the temporomandibular joint $\cap$ Temporomandibular Disorders

• Mary Poppins $\in$ no degeneration of the temporomandibular joint $\cap$ no Temporomandibular Disorders

To arrive at the final diagnosis above, we conducted a probabilistic-causal analysis of Mary Poppins' health status whose initial data were $D=\{\delta _{1},.....\delta _{n}\}$.

In general, we can refer to a logical process in which we examine the following elements:

• an individual: $a$
• its initial data set $D=\{\delta _{1},.....\delta _{n}\}$
• a population sample $n$ to which it belongs,
• a base probability $P(D)=0,003$

At this point we should introduce too specialized arguments that would take the reader off the topic but that have an high epistemic importance for which we will try to extract the most described logical thread of the Analysandum/Analysans concept.

The probabilistic-causal analysis of $D=\{\delta _{1},.....\delta _{n}\}$ is then a couple of the following logical forms (Analysandum / Analysans):

• Analysandum $=\{P(D),a\}$: is a logical form that contains two parameters: probability $P(D)$ to select a person who has the symptoms and elements belonging to the set $D=\{\delta _{1},\delta _{2},...,\delta _{n}\}$, and the generic individual $a$ who is prone to those symptoms.

• Analysan $=\{\pi ,a,KB\}$: is a logical form that contains three parameters: the partition $\pi$, the generic individual $a$ belonging to the population sample $n$ and $KB$ (Knowledge Base) which includes a set of $n>1$ statements of conditioned probability.

For example, it can be concluded that the definitive diagnosis is the following:

$P(D|Deg.TMJ\cap TMDs)=0.95$ - this means that our Mary Poppins is 95% affected by TMDs, since she has a degeneration of the Temporomandibular Joint in addition to the positive data $D=\{\delta _{1},.....\delta _{n}\}$

Final considerations

We took a long and tortuous path to better understand the complexity encountered by the colleague struggling with the very heavy ethical responsibility of making a diagnosis. However, this task becomes even more complex when we need to be detailed and careful in making a differential diagnosis.

Here, we enter a delicate topic, that is connected with the epistemological contents and that first of all was reported in the "Introduction". We are talking about:

• Interdisciplinarity:
  In science policy, it is generally recognized that science-based problem solving requires interdisciplinary research (IDR), as proposed by the EU project called Horizon 2020. In a recent study, the authors focus on the question why researchers have cognitive and epistemic difficulties in conducting IDR. It is believed that the loss of philosophical interest in the epistemology of interdisciplinary research is caused by a philosophical paradigm of science called "Physics Paradigm of Science", which prevents recognition of important IDR changes in both the philosophy of science and research.
  The proposed alternative philosophical paradigm, called 'Engineering Paradigm of Science', makes alternative philosophical assumptions about aspects such as the purpose of science, the character of knowledge, the epistemic and pragmatic criteria for the acceptance of knowledge and the role of technological tools. Consequently, scientific researchers need so-called metacognitive scaffolds to assist them in the analysis and reconstruction of how 'knowledge' is constructed in different disciplines.
  In interdisciplinary research, metacognitive scaffolds help interdisciplinary communication analyse and articulate how the discipline builds knowledge

This concept is linked to the previously discussed topic in which the colleague should be aware of his own 'Subjective Uncertainty' (due to a classic logic language 'sick or healthy') and of 'Objective Uncertainty' (due to a probabilistic logic language 'probably sick or probably healthy'). It is not complicated to prove this assertion: the uncertainty we are talking about derives from the fact that the elements, assertions, data, classes and subclasses mentioned and that build the apparatus of the logic of probabilistic's language: Analysandum $=\{P(D),a\}$ and Analysan $=\{\pi ,a,KB\}$ are elements that exist in a specific world, and in this case in a dental context in which the element $KB$ of the process indisputably indicates a "basic knowledge" only in a specific dental context.

This conclusion confirmed by the dentist was the following:

$P(D|Deg.TMJ\cap TMDs)=0.95$

or better: it is my 95% belief that Mary Poppins is affected by TMDs since she has a degeneration of the temporomandibular joint in addition to the positivity of the data $D=\{\delta _{1},\dots \delta _{n}\}$But something strange happens because out of nowhere, a researcher, who uses 'metacognitive scaffolds' for an implementation in the analysis and reconstruction of how 'knowledge' is built in different disciplines, demands an answer to the following question from the dentist:

 

«...is there another world or context, parallel to yours, in which in addition to the D data there are further data unknown to you?»

and increase the dose: submit Mary Poppins to the following trigeminal electrophysiological tests, label them as we did previously for the set data $D=\{\delta _{1},\dots \delta _{n}\}$ generating another set containing a number $m$ of unknown data (not belonging to the purely dental branch) $C=\{\gamma _{1},\dots \gamma _{m}\}$ thereby creating an entirely new set that we will call $S_{unknow}=D+C=\{\delta _{1},\dots ,\delta _{n},\gamma _{1},\dots ,\gamma _{m}\}$ (called $S_{unknown}$ precisely due to the presence of data unknown to the dental context).

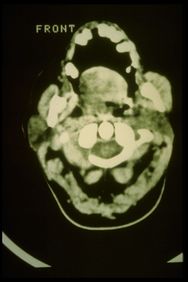
$\delta _{1}=$ Positive radiological report of the TMJ in Figure 2

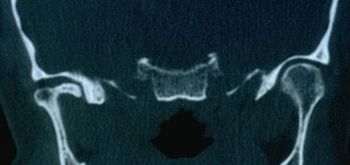
$\delta _{2}=$ Positive CT report of the TMJ in Figure 3

$\delta _{3}=$ Positive axiographic report of the condylar traces in Figure 4

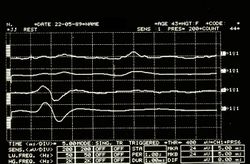
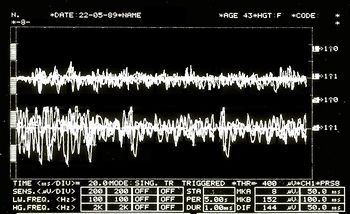
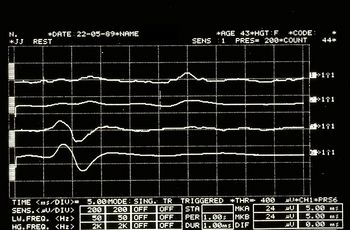
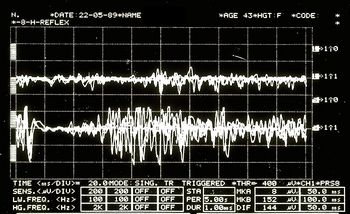
$\delta _{4}=$ Asymmetric EMG interference pattern in Figure 5

${\gamma _{1}}=$ Jaw jerk in Figure 6

${\displaystyle \gamma _{2}}=$ Mechanical Silent Period in Figure 7

${\displaystyle \gamma _{3}}=$ CT right masseter muscle in Figure 8

Third Clinical Approach

(hover over the images)

• 

  Figure 1: Patient reporting "Orofacial pain in the right hemilateral)

• 

  Figure 2: Patient's TMJ Stratigraphy showing signs of condylar flattening and osteophyte

• 

  Figure 3: Computed Tomography of the TMJ

• 

  Figure 4: Axiography of the patient showing a flattening of the chewing pattern on the right condyle

• 

  Figure 5: EMG Interferential Pattern. Overlapping upper traces corresponding to the right masseter, lower to the left masseter.

• 

  Figure 6: Jaw jerk electrophysiologically detected on the right (upper traces) and left (lower traces) masseters

• 

  Figure 7: Mechanical silent period detected electrophysiologically on the right (upper overlapping traces) and left (lower overlapping traces) masseters

• 

  Figure 8: Axial CT of the facial massif in which there is a marked hypertrophy of the right masseter

In this way it has been shown that, inevitably,

«the logic of medical language is more or less based on data that derive from a specific world or context or rather, a specialistic context in which the perimeter that delimits this knowledge does not allow us to project ourselves into parallel contexts»

By exploring this perimeter line of the specialist context, we will create an area close to it which we will call the 'fuzzy zone' or 'fuzzy logic' which we will discuss in the next chapter.

 

«... from what it seems not even with a probabilistic language logic we will be able to define an exact diagnosis.» (in fact, for this reason we should also consider Fuzzy Logic Language)

Bibliography & references

Masticationpedia is a charity, a not-for-profit organization operating in dentistry medical research,
particularly focusing on the field of the neurophysiology of the masticatory system
 

Home

Introduction

Latest chapter

Ed. Lab

Donate

Contacts