Difference between revisions of "Store:FLes05"

Latest revision as of 19:02, 13 March 2023

Conjunto difuso ${\tilde {A}}$ y función de pertenencia $\mu _{\displaystyle {\tilde {A}}}(x)$

Elegimos, como formalismo, representar un conjunto borroso con la 'tilde': ${\tilde {A}}$ Un conjunto borroso es un conjunto donde los elementos tienen un 'grado' de pertenencia (de acuerdo con la lógica borrosa): algunos pueden incluirse en el conjunto en 100%, otros en porcentajes menores.

Para representar matemáticamente este grado de pertenencia se encuentra la función $\mu _{\displaystyle {\tilde {A}}}(x)$ denominada 'Función de pertenencia'. La función $\mu _{\displaystyle {\tilde {A}}}(x)$ es una función continua definida en el intervalo $[0;1]$ donde es:

$\mu _{\tilde {A}}(x)=1\rightarrow$ si $x$ está totalmente contenido en $A$ (estos puntos se llaman 'núcleo', indican valores predicados plausibles).
$\mu _{\tilde {A}}(x)=0\rightarrow$ si $x$ no está contenido en $A$
$0<\mu _{\tilde {A}}(x)<1\;\rightarrow$ si $x$ está parcialmente contenido en $A$ (estos puntos se llaman 'soporte', indican los posibles valores predicados).

La representación gráfica de la función $\mu _{\displaystyle {\tilde {A}}}(x)$ puede ser variado; desde los de líneas lineales (triangulares, trapezoidales) hasta los que tienen forma de campana o 'S' (sigmoidales) como se muestra en la Figura 1, que contiene todo el concepto gráfico de la función de pertenencia....^[1]^[2]

Figure 1: Types of graphs for the membership function.

El conjunto soporte de un conjunto borroso se define como la zona en la que el grado de pertenencia resulta $0<\mu _{\tilde {A}}(x)<1$ ; por su parte, el núcleo se define como el ámbito en el que el grado de pertenencia asume el valor $\mu _{\tilde {A}}(x)=1$

El 'Conjunto de soporte' representa los valores del predicado que se consideran posibles, mientras que el 'núcleo' representa los que se consideran más plausibles.

Si ${A}$ representara un conjunto en el sentido ordinario del término o en la lógica del lenguaje clásico descrita anteriormente, su función de pertenencia podría asumir solo los valores $1$ o $0$ , $\mu _{\displaystyle {A}}(x)=1\;\lor \;\mu _{\displaystyle {A}}(x)=0$ finalizando en si el elemento $x$ pertenece o no al todo, según se considere. La Figura 2 muestra una representación gráfica del concepto nítido (rígidamente definido) o confuso de membresía, que recuerda claramente las consideraciones de Smuts.^[3]

Volvamos al caso concreto de nuestra Mary Poppins, en el que vemos una discrepancia entre las afirmaciones del dentista y del neurólogo y buscamos una comparación entre la lógica clásica y la lógica difusa:

Figure 2: Representation of the comparison between a classical and fuzzy ensemble.

Figura 2: Imaginemos el Universo de la Ciencia $U$ en el que existen dos mundos o contextos paralelos, el ${A}$ y el ${\tilde {A}}$

${A}=$ En el contexto científico, el llamado ‘crisp’, y lo hemos convertido a la lógica del Lenguaje Clásico, en el que el médico dispone de una información científica absoluta $KB$ con una clara línea divisoria que hemos denominado $KB_{c}$

${\tilde {A}}=$ En otro contexto científico llamado ‘lógica difusa’, y en el que existe una unión entre el subconjunto ${A}$ en ${\tilde {A}}$ que podemos llegar a decir: unión entre $KB_{c}$

Notaremos notablemente las siguientes deducciones:

Lógica Clásica en el Contexto Odontológico ${A}$ en el que sólo será posible un proceso lógico que dé como resultado $\mu _{\displaystyle {A}}(x)=1$ , o siendo $\mu _{\displaystyle {A}}(x)=0$ el rango de datos $D=\{\delta _{1},\dots ,\delta _{4}\}$ reducido a conocimientos básicos $KB$ en el conjunto ${A}$ . Esto quiere decir que fuera del mundo odontológico existe un void y ese término de la teoría de conjuntos se escribe precisamente $\mu _{\displaystyle {A}}(x)=0$ y que es sinónimo de un rango alto de:

«Error de diagnóstico diferencial»

Lógica difusa en un contexto dental ${\tilde {A}}$ en el que se representan más allá de los conocimientos básicos $KB$ del contexto dental también aquellos parcialmente adquiridos del mundo neurofisiológico $0<\mu _{\tilde {A}}(x)<1$ tendrán la prerrogativa de devolver un resultado $\mu _{\tilde {A}}(x)=1$ y un resultado $0<\mu _{\tilde {A}}(x)<1$ debido a conocimientos básicos $KB$ que en este punto está representado por la unión de $KB_{c}$ contextos dentales y neurológicos. El resultado de esta implementación científico-clínica de la odontología permitiría una
«Reducción del error de diagnóstico diferencial»

↑ Zhang W, Yang J, Fang Y, Chen H, Mao Y, Kumar M, «Analytical fuzzy approach to biological data analysis», in Saudi J Biol Sci, 2017».
PMID:28386181 - PMCID:PMC5372457
DOI:10.1016/j.sjbs.2017.01.027
↑ Lazar P, Jayapathy R, Torrents-Barrena J, Mol B, Mohanalin, Puig D, «Fuzzy-entropy threshold based on a complex wavelet denoising technique to diagnose Alzheimer disease», in Healthc Technol Lett, The Institution of Engineering and Technology, 2016».
PMID:30800318 - PMCID:PMC6371778
DOI:10.1049/htl.2016.0022
↑ •SMUTS J.C. 1926, Holism and Evolution, London: Macmillan.

[1] Zhang W, Yang J, Fang Y, Chen H, Mao Y, Kumar M, «Analytical fuzzy approach to biological data analysis», in Saudi J Biol Sci, 2017».
PMID:28386181 - PMCID:PMC5372457
DOI:10.1016/j.sjbs.2017.01.027

[2] Lazar P, Jayapathy R, Torrents-Barrena J, Mol B, Mohanalin, Puig D, «Fuzzy-entropy threshold based on a complex wavelet denoising technique to diagnose Alzheimer disease», in Healthc Technol Lett, The Institution of Engineering and Technology, 2016».
PMID:30800318 - PMCID:PMC6371778
DOI:10.1049/htl.2016.0022

[:0-3] •SMUTS J.C. 1926, Holism and Evolution, London: Macmillan.

[1]

[2]

[3]

@@ Line 1: / Line 1: @@
-==Fuzzy set <math>\tilde{A}</math> and membership function <math>\mu_{\displaystyle {\tilde {A}}}(x)</math>==
+==Conjunto difuso <math>\tilde{A}</math> y función de pertenencia <math>\mu_{\displaystyle {\tilde {A}}}(x)</math>==
-We choose - as a formalism - to represent a fuzzy set with the 'tilde':<math>\tilde{A}</math>. A fuzzy set is a set where the elements have a 'degree' of belonging (consistent with fuzzy logic): some can be included in the set at 100%, others in lower percentages.
+Elegimos, como formalismo, representar un conjunto borroso con la 'tilde': <math>\tilde{A}</math> Un conjunto borroso es un conjunto donde los elementos tienen un 'grado' de pertenencia (de acuerdo con la lógica borrosa): algunos pueden incluirse en el conjunto en 100%, otros en porcentajes menores.
-To mathematically represent this degree of belonging is the function <math>\mu_{\displaystyle {\tilde {A}}}(x)</math> called ''''Membership Function''''. The function <math>\mu_{\displaystyle {\tilde {A}}}(x)</math> is a continuous function defined in the interval <math>[0;1]</math>where it is:
+Para representar matemáticamente este grado de pertenencia se encuentra la función <math>\mu_{\displaystyle {\tilde {A}}}(x)</math> denominada ''''Función de pertenencia'''<nowiki/>'. La función <math>\mu_{\displaystyle {\tilde {A}}}(x)</math> es una función continua definida en el intervalo <math>[0;1]</math> donde es:
-*<math>\mu_ {\tilde {A}}(x) = 1\rightarrow </math> if <math>x</math> is totally contained in <math>A</math> (these points are called 'nucleus', they indicate <u>plausible</u> predicate values).
+*<math>\mu_ {\tilde {A}}(x) = 1\rightarrow </math> si <math>x</math> está totalmente contenido en <math>A</math> (estos puntos se llaman ''''núcleo'''<nowiki/>', indican valores predicados <u>plausibles</u>).
-*<math>\mu_ {\tilde {A}}(x) = 0\rightarrow </math> if <math>x</math> is not contained in <math>A</math>
+*<math>\mu_ {\tilde {A}}(x) = 0\rightarrow </math>si <math>x</math> no está contenido en <math>A</math>
-*<math>0<\mu_ {\tilde {A}}(x) < 1 \;\rightarrow </math> if <math>x</math> is partially contained in <math>A</math> (these points are called 'support', they indicate the <u>possible</u> predicate values).
+*<math>0<\mu_ {\tilde {A}}(x) < 1 \;\rightarrow </math>si <math>x</math> está parcialmente contenido en <math>A</math> (estos puntos se llaman ''''soporte'''<nowiki/>', indican los <u>posibles</u> valores predicados).
-The graphical representation of the function <math>\mu_{\displaystyle {\tilde {A}}}(x)</math> can be varied; from those with linear lines (triangular, trapezoidal) to those in the shape of bells or 'S' (sigmoidal) as depicted in Figure 1, which contains the whole graphic concept of the function of belonging.<ref>{{Cite book
+La representación gráfica de la función <math>\mu_{\displaystyle {\tilde {A}}}(x)</math> puede ser variado; desde los de líneas lineales (triangulares, trapezoidales) hasta los que tienen forma de campana o 'S' (sigmoidales) como se muestra en la Figura 1, que contiene todo el concepto gráfico de la función de pertenencia....<ref>{{Cite book
   | autore = Zhang W
   | autore2 = Yang J
@@ Line 53: / Line 53: @@
 [[File:Fuzzy_crisp.svg|alt=|left|thumb|400px|'''Figure 1:''' Types of graphs for the membership function.]]
-The '''support set''' of a fuzzy set is defined as the zone in which the degree of membership results <math>0<\mu_ {\tilde {A}}(x) < 1</math>; <!--131-->on the other hand, the '''core''' is defined as the area in which the degree of belonging assumes value <math>\mu_ {\tilde {A}}(x) = 1</math>
+El '''conjunto soporte''' de un conjunto borroso se define como la zona en la que el grado de pertenencia resulta <math>0<\mu_ {\tilde {A}}(x) < 1</math>; por su parte, el '''núcleo''' se define como el ámbito en el que el grado de pertenencia asume el valor <math>\mu_ {\tilde {A}}(x) = 1</math>
-The 'Support set' represents the values of the predicate deemed '''possible''', while the 'core' represents those deemed more '''plausible'''.
+El ''''Conjunto de soporte'''<nowiki/>' representa los valores del predicado que se consideran '''posibles''', mientras que el 'núcleo' representa los que se consideran más '''plausibles'''.
-If <math>{A}</math> <!--134-->represented a set in the ordinary sense of the term or classical language logic previously described, its membership function could assume only the values <math>1</math> <!--135-->or <math>0</math>, <math>\mu_{\displaystyle {{A}}}(x)= 1 \; \lor \;\mu_{\displaystyle {{A}}}(x)= 0</math> <!--136-->depending on whether the element <math>x</math> <!--137-->belongs to the whole or not, as considered. <!--138-->Figure 2 shows a graphic representation of the crisp (rigidly defined) or fuzzy concept of membership, which clearly recalls Smuts's considerations.<ref name=":0">•SMUTS J.C. 1926, [[wikipedia:Holism_and_Evolution|<!--139-->Holism and Evolution]], London: Macmillan.</ref>
+Si <math>{A}</math> representara un conjunto en el sentido ordinario del término o en la lógica del lenguaje clásico descrita anteriormente, su función de pertenencia podría asumir solo los valores <math>1</math> o <math>0</math>, <math>\mu_{\displaystyle {{A}}}(x)= 1 \; \lor \;\mu_{\displaystyle {{A}}}(x)= 0</math> finalizando en si el elemento <math>x</math> pertenece o no al todo, según se considere. La Figura 2 muestra una representación gráfica del concepto nítido (rígidamente definido) o confuso de membresía, que recuerda claramente las consideraciones de Smuts.<ref name=":0">•SMUTS J.C. 1926, [[wikipedia:Holism_and_Evolution|<!--139-->Holism and Evolution]], London: Macmillan.</ref>
-Let us go back to the specific case of our Mary Poppins, in which we see a discrepancy between the assertions of the dentist and the neurologist and we look for a comparison between classical logic and fuzzy logic:
+Volvamos al caso concreto de nuestra Mary Poppins, en el que vemos una discrepancia entre las afirmaciones del dentista y del neurólogo y buscamos una comparación entre la lógica clásica y la lógica difusa:
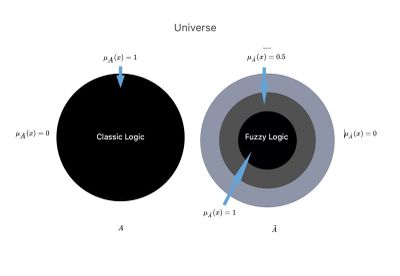
-[[File:Fuzzy1.jpg|thumb|400x400px|'''<!--141-->Figure 2:''' <!--142-->Representation of the comparison between a classical and fuzzy ensemble.]]
+[[File:Fuzzy1.jpg|thumb|400x400px|'''Figure 2:''' Representation of the comparison between a classical and fuzzy ensemble.]]
-'''Figure 2:''' Let us imagine the Science Universe <math>U</math> <!--145-->in which there are two parallel worlds or contexts, <math>{A}</math> <!--146-->and <math>\tilde{A}</math>.
+Figura 2: Imaginemos el Universo de la Ciencia <math>U</math> en el que existen dos mundos o contextos paralelos, el <math>{A}</math> y el <math>\tilde{A}</math>
-<math>{A}=</math>  In the scientific context, the so-called ‘crisp’, and we have converted into ''the logic'' of ''Classic Language'', in which the physician has an absolute scientific background information <math>KB</math>  <!--148-->with a clear dividing line that we have named <math>KB_c</math>.
-<math>\tilde{A}=</math> In another scientific context called  ‘fuzzy logic’, and in which there is a union between the subset <math>{A}</math> <!--150-->in <math>\tilde{A}</math> <!--151-->that we can go so far as to say: union between <math>KB_c</math>.
+<math>{A}=</math> En el contexto científico, el llamado ‘crisp’, y lo hemos convertido a [[la lógica del Lenguaje Clásico]], en el que el médico dispone de una información científica absoluta <math>KB</math> con una clara línea divisoria que hemos denominado <math>KB_c</math>
-We will remarkably notice the following deductions:
-*'''Classical Logic''' in the Dental Context <math>{A}</math> in which only a logical process that gives as results <math>\mu_{\displaystyle {{A}}}(x)= 1 </math> will be possible, or <math>\mu_{\displaystyle {{A}}}(x)= 0 </math> being the range of data <math>D=\{\delta_1,\dots,\delta_4\}</math> reduced to basic knowledge <math>KB</math> in the set <math>{A}</math>. This means that outside the dental world there is a void and that term of set theory is written precisely <math>\mu_{\displaystyle {{A}}}(x)= 0 </math> and which is synonymous with a high range of:
+<math>\tilde{A}=</math> En otro contexto científico llamado ‘[[lógica difusa]]’, y en el que existe una unión entre el subconjunto <math>{A}</math> en <math>\tilde{A}</math> que podemos llegar a decir: unión entre <math>KB_c</math>
-{{q2|Differential diagnostic error|}}
-*'''Fuzzy logic''' in a dental context <math>\tilde{A}</math> in which they are represented beyond the basic knowledge <math>KB</math> of the dental context also those partially acquired from the neurophysiological world <math>0<\mu_ {\tilde {A}}(x) < 1</math> will have the prerogative to return a result <math>\mu_\tilde{A}(x)= 1
+Notaremos notablemente las siguientes deducciones:
-  </math> and a result <math>0<\mu_ {\tilde {A}}(x) < 1</math> because of basic knowledge <math>KB</math> which at this point is represented by the union of <math>KB_c</math> dental and neurological contexts. The result of this scientific-clinical implementation of dentistry would allow a {{q2|Reduction of differential diagnostic error|}}
+*'''Lógica Clásica''' en el Contexto Odontológico <math>{A}</math> en el que sólo será posible un proceso lógico que dé como resultado <math>\mu_{\displaystyle {{A}}}(x)= 1 </math>, o siendo <math>\mu_{\displaystyle {{A}}}(x)= 0 </math> el rango de datos <math>D=\{\delta_1,\dots,\delta_4\}</math> reducido a conocimientos básicos <math>KB</math> en el conjunto <math>{A}</math>. Esto quiere decir que fuera del mundo odontológico existe un void y ese término de la teoría de conjuntos se escribe precisamente <math>\mu_{\displaystyle {{A}}}(x)= 0 </math> y que es sinónimo de un rango alto de:
+{{q2|Error de diagnóstico diferencial|}}
+*'''Lógica difusa''' en un contexto dental <math>\tilde{A}</math> en el que se representan más allá de los conocimientos básicos <math>KB</math> del contexto dental también aquellos parcialmente adquiridos del mundo neurofisiológico <math>0<\mu_ {\tilde {A}}(x) < 1</math> tendrán la prerrogativa de devolver un resultado <math>\mu_\tilde{A}(x)= 1
+  </math> y un resultado <math>0<\mu_ {\tilde {A}}(x) < 1</math> debido a conocimientos básicos <math>KB</math> que en este punto está representado por la unión de <math>KB_c</math> contextos dentales y neurológicos. El resultado de esta implementación científico-clínica de la odontología permitiría una {{q2|Reducción del error de diagnóstico diferencial|}}

Difference between revisions of "Store:FLes05"

Latest revision as of 19:02, 13 March 2023

Conjunto difuso A ~ {\displaystyle {\tilde {A}}} y función de pertenencia μ A ~ ( x ) {\displaystyle \mu _{\displaystyle {\tilde {A}}}(x)}

Conjunto difuso ${\tilde {A}}$ y función de pertenencia $\mu _{\displaystyle {\tilde {A}}}(x)$